Despite conscientious attempts to equilibrate vibratory variables such as bowl amplitude, roll angle, media species, media volume, part loading, process liquid concentration and flow rate, what appear to be identically set-up vibratory bowls will nonetheless finish identical loads of parts in varying time cycles.
 William NebioloWhy is this so? This paper will explore this phenomenon. Techniques will be introduced that will allow operators to capture operational characteristics that aren’t typically apparent. A formula will then be introduced that will allow operators to apply this new data to calculate the amount of force that the media is actually applying to the parts.
William NebioloWhy is this so? This paper will explore this phenomenon. Techniques will be introduced that will allow operators to capture operational characteristics that aren’t typically apparent. A formula will then be introduced that will allow operators to apply this new data to calculate the amount of force that the media is actually applying to the parts.
It is the efficiency of the force applied to the parts during vibratory finishing that controls the efficiency and speed of the refinement cycle.
What is Vibratory Force?
During vibratory bowl processing, the bowl’s operating channel is converted into a fluidized bed of deburring media. Vibratory media is the tool that is used to either deburr or polish the parts placed into the machine.
The applied force this media can generate on the parts can be partially predicted using Newton’s Second Law; F = ma, where the variable “m”, equates to mass.1 In this paper “m” will be considered to be the weight of the media above the part at mid-channel.
The variable “a” equates to the acceleration of the mass.1 In other words, an accelerating media mass will apply a greater finishing force to the part’s surface as compared to a slower moving equivalent weight of media. The formula F = ma will be incorporated into a new formula that will be introduced later in this paper. When measurable process variables are inserted into the new formula a better understanding of efficiency differences between apparently, identically operating vibratory bowls can be calculated.
How is “M”; Mass, Determined?
 Image No. 1: mass is the weight of the media column above a part at the bottom of the bowl at mid-channel.Mass is the easiest variable to determine and in this paper is considered to be the weight; in pounds, of the media column, above the part, at mid-channel. See Image 1.
Image No. 1: mass is the weight of the media column above a part at the bottom of the bowl at mid-channel.Mass is the easiest variable to determine and in this paper is considered to be the weight; in pounds, of the media column, above the part, at mid-channel. See Image 1.
The poundage of mass is affected by:
- Available depth of the vibe bowl channel
- Attrition induced media depth reduction
- Weight density of the media being used
All types of vibratory media are affected by media attrition. With each passing hour a certain weight percentage of media is lost to frictional abrasion. It exits the bowl as swarf in the effluent flow stream.4, 5, 6Therefore; microscopically, each individual piece of media, decreases in volume every hour.5, 6 Media attrition is most noticeable as a decrease in the height of media in the vibe bowl’s channel over time.
As the volume of media decreases, so too does the weight in pounds of media above the part at bowl mid-channel. See image No. 2 below.6
 Image No. 2: At left, image6 of a vibratory bowl with the proper depth of media present in the bowl channel. At right, image6 of the same vibratory bowl where the media depth is too low, caused by attrition
Image No. 2: At left, image6 of a vibratory bowl with the proper depth of media present in the bowl channel. At right, image6 of the same vibratory bowl where the media depth is too low, caused by attrition
Image No. 2 shows a properly loaded vibratory bowl channel.6 The channel has an available depth of 10” and it contains at proper loading, 10” of media. This will allow the operator optimal machine performance relative to media volume. The right image shows the same vibe bowl after a prolonged period of running without adding media.6 The depth of media now measures just 6”. With 4” of media lost to attrition, approximately 40% of the media volume is now missing. As a result, the weight in pounds of media in the column above the part is reduced comparably. If the reduced poundage of media in image No. 3 is entered into the formula F = ma, then the amount of force or “F” that the column of media above the part can apply is also reduced. Less applied force means more required more time to accomplish the same amount of work.
In vibratory finishing the species of media utilized is dependent upon the desired finish requirement for the parts and the speed with which the operation is to be performed.5, 6 (See Table No. 1 below.)
Table No. 1; Media Comparison Table
| Media Type | Density lbs/ft3 | Substrate to be Deburred or Polished |
| HDNA1 | 125 | Rc > 40 Ht. Tr’d. Steel |
| Porcelain | 90 | Non-HT Steel no burrs |
| Ab. Cer.2 #10 | 88 | Steel light burrs |
| Ab. Cer. #20 | 85 | Steel moderate burrs |
| Ab. Cer. #30 | 82 | Steel medium burrs |
| Ab. Cer. #40 | 80 | Steel heavy burrs |
| Ab. Cer. #50 | 78 | Steel very heavy burrs |
| Poly. Plas.3 | 65 | Al; Brass; Cu & Zn |
1 HDNA; High-Density Non-Abrasive; 2 Ab. Cer.; Abrasive Ceramic; 3 Polyester Plastic
A high-density, non-abrasive media is typically used for chemically accelerated finishing of hardened steel parts.5, 6 Abrasive ceramic media is generally used for generic part deburring.5, 6 Lighter-density, plastic media is used to minimize media impingement damage on metallurgically softer metals such as aluminum, brass, copper and zinc.5, 6
Vibratory bowls are sold by their volumetric displacement. Typically, larger volume machines have deeper channels and will hold more pounds of media. If we examine a small area at the bottom of the vibe bowl’s operating channel, a machine with a deep channel will hold a taller column of media mass above that same area. More media mass means more pounds of media and more “m”. The larger the “m” the more force is applied to a part’s surface. Consider as an example a submarine. (See Images No. 3 below.)
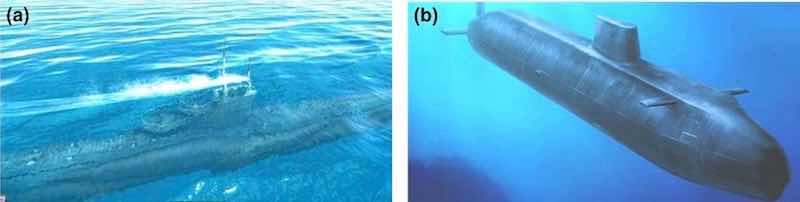 Image No. 3: at left, a submarine near the surface has little water mass above its hull because the column of water above the submarine is virtually nonexistent. At right, a submarine at depth has significant water mass on its hull because the column of water above it is vast.
Image No. 3: at left, a submarine near the surface has little water mass above its hull because the column of water above the submarine is virtually nonexistent. At right, a submarine at depth has significant water mass on its hull because the column of water above it is vast.
The deeper the submarine dives, the more water pressure is applied to its hull, because there are more pounds of water above it.
Mass Rolling Velocity
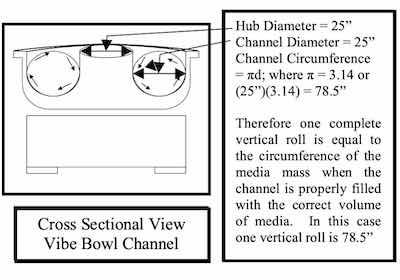 Image No. 4; this is a 45ft3 vibe bowl capable of holding 36ft3 of media. It has a 25” wide channel. Vertical roll is 78.5” or the circumference of the 25” diameter circle formed by the media mass in the bowl’s channel, when properly filled with media.3,6Mass rolling velocity can be measured as the distance a part travels per minute of time. The further the distance traveled per minute of time, the more work that is done in that minute of time because the part is contacted by the media more often.3
Image No. 4; this is a 45ft3 vibe bowl capable of holding 36ft3 of media. It has a 25” wide channel. Vertical roll is 78.5” or the circumference of the 25” diameter circle formed by the media mass in the bowl’s channel, when properly filled with media.3,6Mass rolling velocity can be measured as the distance a part travels per minute of time. The further the distance traveled per minute of time, the more work that is done in that minute of time because the part is contacted by the media more often.3
Mass movement in the vibratory bowl undergoes two, simultaneous planes of motion; vertical roll and horizontal slide.3, 6 Vertical roll is shown in image No. 4.
Vertical roll is the circumference of the circle formed where the width of the channel is the circle diameter.3, 6 A vibe bowl channel properly loaded with media will generate maximum circumference.
When media level is low due to attrition, the circle diameter is smaller, its resulting circumference is shorter and so too is the mass rolling distance. Horizontal slide is the distance required to lap the bowl channel one time.3, 6 See Image No. 5.
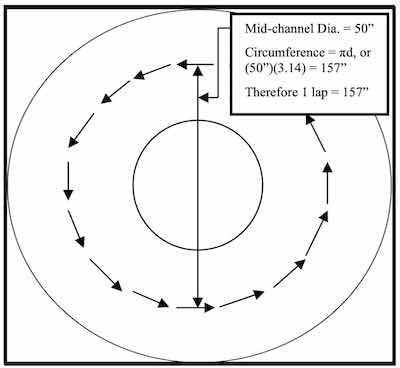 Figure 5 - An overhead view of the 45 ft3 vibratory bowl shown in Fig. 4, illustrating the concept of horizontal slide.
Figure 5 - An overhead view of the 45 ft3 vibratory bowl shown in Fig. 4, illustrating the concept of horizontal slide.
This circle is considered the average horizontal distance traveled because half the time parts are on the outside of this line and half the time they are on the inside of this line. Horizontal distance traveled for Image No. 7 is calculated at 157”.3, 6
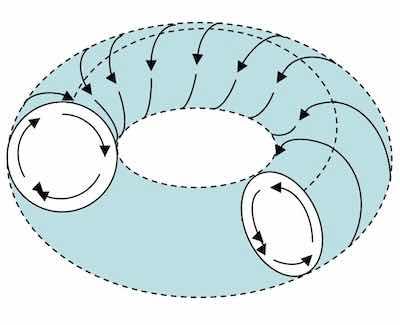 Figure 6 - The mass helical motion pattern when vertical roll and horizontal slide are combined.6
Figure 6 - The mass helical motion pattern when vertical roll and horizontal slide are combined.6
Combining vertical roll and horizontal slide, results in a helical, spiral, mass-motion pattern. See Image No. 8 below.
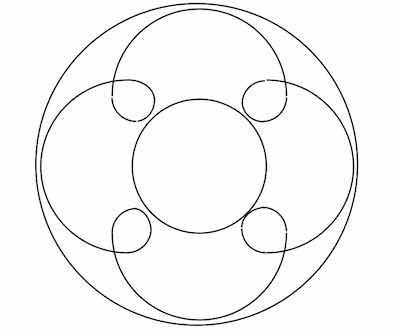 Figure 7 - The helical motion pattern of four vertical rolls per horizontal lap in 60 seconds time.
Figure 7 - The helical motion pattern of four vertical rolls per horizontal lap in 60 seconds time.
Image No. 8; shows the mass helical motion pattern when vertical roll and horizontal slide are combined.6
Calculation of Mass Velocity
Using the circumference information just described for vertical roll and horizontal slide, it’s possible to calculate mass velocity as the distance parts travel per minute of processing time. Being able to complete this calculation is important to being able to later calculate applied media force.
As an example, let us assume that a part placed in the 45ft3 vibratory bowl, makes four vertical rolls per one horizontal lap and that this action is completed in 60 seconds. This motion is show in Image No. 9 below.6
Image No. 9; shows a helical motion pattern of 4 vertical rolls per 1 horizontal lap in 60 seconds time.
It is possible to calculate the part’s velocity or distance traveled per minute as follows:
- Vertical = (4)(78.5”) = 314”
- Horizontal = (1)(157”) = +157”
- Distance in 1 minute = 471”
Projecting Part Contact Area
During vibratory processing, parts will move through and be contacted by the media mass orthographically.6 During orthographic roll, a part; regardless of size and shape will rotate about its x, y and z axis within a sphere of volume inscribed by its longest; or length, dimension.6 See Image No. 10, next column.6
At any one moment in time during vibratory processing, the orthographic rotational alignment of the part relative to its x, y or z axes is random and indiscriminant. We can assume however, that for the overall duration of the process cycle, its alignment to the media mass will be uniformly distributed relative to its x, y and z axes.
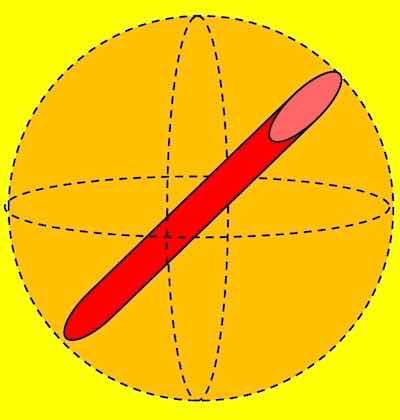 Figure 8 - Orthographic representation shows the sphere of volume within which the red cylinder rotates along its x, y and z axes.6
Figure 8 - Orthographic representation shows the sphere of volume within which the red cylinder rotates along its x, y and z axes.6
Since media force is applied to an area of the part, the question arises; “What one area of the part is being contacted at any one particular moment in time?” This is an unknown entity as we can’t see the momentary alignment of the part within the media mass at any one moment and therefore the area contacted is an indeterminable variable.
However, it is possible to calculate a “part’s average area.” In orthographic rotation for the entire processing cycle the average area is what will be forcefully contacted by the media at any moment in time.
Illustrative Example; Average Part Area
Here is an example. Assume the red cylinder in Image No. 10 is a part 8” in length, 1” in width and 2” in height. Its average area can be determined by volumetric dimensions from the smallest box which envelops it, if it is placed flat on a table as follows:
Average Part Area Calculation
- length 1; “x” axis area = (1”)(8”) = 8in2
- length 2; “x” axis area = (2”)(8”) = 16in2
- Σ length area = (side 1 + side 2)(2) = 48in2
- length area = 48 in2÷ 4 “x” axes = 12in2
- end 1; “y” axis area = (1”)(2”) = 2in2
- end 2; “z” axis area = (2”)(1”) = + 2in2
- Total x + y + z area = 16in2
- Area = 16in2÷ 3 axes = 5.33in2
So we can say that on average 5.33in2 of the part is being forcefully contacted by media at any one moment in time. This same model for calculating the average surface area of any shaped part can be used. Simply take the dimensions of the smallest rectangular box that will envelop the part.
Average Surface Area Assumptions
Note we must make two assumptions for the average area calculation above. They are:
Assumption One: An ornately configured part will have an exact area that may be somewhat larger or smaller than the area of the box within which it can be contained. Even though this may be true, using the average area and not the exact area will be sufficient for comparing the efficiencies of identical machines in the vibratory processing room.
Assumption Two: The volume of the box enveloping the part has 4-each, x-axis or length sides. By taking the average length area of the sum of the two narrow and two wide sides we can determine the average length area exposed to the media when the part is in the x-axis-orientation at the bowl’s mid-channel base.
The Effect of Centrifugal Force
Centrifugal force via centrifugal barrel and centrifugal disc units reduce processing time4, and this equipment is commonly found in finishing departments. See Images No. 11 and No. 12 below.4, 6
 Figure 9 - (a) A centrifugal barrel finisher; (b) a centrifugal disc finisher.
Figure 9 - (a) A centrifugal barrel finisher; (b) a centrifugal disc finisher.
In a traditional vibratory bowl, a very slight centrifugal force, is generated in the axial portion of the mass.6 See Image No. 136.
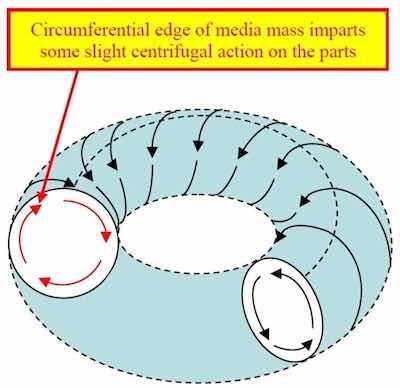 Figure 10 - Mass rolling action and location of the axial centrifugal force.6
Figure 10 - Mass rolling action and location of the axial centrifugal force.6
The traditional formula for centrifugal force is given as F = mv2/r. By taking into consideration the force of gravity on the average surface area of the part by the column of media above it and combining the traditional mechanical engineering force formula of F = ma with the formula for centrifugal force we can generate a new formula that can be used to determine the force being applied to the average surface area of a part during vibratory processing. The new media contact force formula so created is shown as: Media Contact Force Formula: MCF = (m/g)(v2/r)
Where formula variables are:
- m = lbs of media column above av. area
- g = gravity; a constant of 32 ft/sec2
- v = part velocity in feet per second
- r = radius of the media mass present
This formula is useful because it allows the vibratory operator to understand more fully how the following variables affect vibe bowl performance:
- Different media having different densities
- Varying velocity; fast bowl vs. slow bowl
- Different bowls with different channel radii
- Attrition induced varying media radii
Determining the MCF Variables
There are four variables which must be substituted into the MCF Formula, to determine media contact force. They are determined as follows: m = Mass of Media Variable
To calculate the lbs. of applied media mass above the part, we will use as an example:
- The density of HDNA media as found in Table No. 1 on page 3.
- The average part area of the part as calculated on page 6.
- The 45ft3vibe bowl containing; when properly loaded, 36ft3of media as shown in images No. 6 and 7 on page 4.
The media applies a force equal to the weight in pounds of the column of media above the average surface area of the part. For this and all subsequent calculations using the MCF formula it will be assumed that the part is centered at the base of the channel, congruent with the channel bisecting circle. See image No. 14 below.
 Figure 11 - The column of media above part average surface area.
Figure 11 - The column of media above part average surface area.
Determine the weight in lbs of the media column above the average part surface area:
- Weight of mass in lbs
- From Table 1; 1ft3HDNA = 125 lbs/ft3
- area of part from page 6 = 5.33in2
- of column of media above av. part area:
- (5.33in2)(25”) = 133.25in3
- 25in3÷ 1,728in3/ft3= 0.077ft3
- lbs mass = (0.077ft3)(125lbs/ft3) = 9.63 lbs
V = Velocity of Part Variable
We will use as part velocity, the velocity of 471” per min. as determined on page 5. This velocity must be converted into feet per second as follows:
- 471 in/min ÷ 12 in/ft = 39.25ft/min
- 25ft/min ÷ 60 sec/min = 0.654ft/sec
Establishing a Standard Control Model for the Media Contact Force Calculation
The Standard Control Model for this example or any vibratory room, is the ideally set-up vibratory bowl. That is, the vibe bowl that has the optimum velocity, the optimum media density and the optimum media level for the processing cycles being used. Substituting the variables just determined into the MCF Formula, we will establish a standard control model for this paper. This model then becomes the standard against which other bowls are compared or the standard against which the bowl itself is compared in the future as operating conditions change. The MCF formula will be utilized to determine the lbs of media force applied to the average part surface area during finishing.
MCF Variables for Standard Control:
- 45ft3 bowl; channel diameter of 25” = 25 inches ÷ 12 inches/ft = 2.08ft
- Channel radius = 2.08ft ÷ 2 = 1.04ft
- Gravity = 32ft/sec2
- Mass of applied media = 9.63 lbs
- Mass velocity = 0.654ft/sec
MCF Calculations for Standard Control:
- Where; MCF = (m/g)(v2/r)
- = [9.63 lb ÷ 32ft/sec2][(0.654 ft/sec)2÷ 1.04ft]
- = (0.30 lbs/ft/sec2) [(0.43ft2/sec2÷ 1.04ft)]
- = (0.30 lbs/ft/sec2)(0.41ft/sec2)
- MCF = 0.123 lbs of media contact force
To understand how this is relevant to the performance of similar vibratory bowls in the same vibratory department, let’s now use the MCF to calculate the lbs. of applied media force in three comparative examples that are relevant to normal vibratory room operating conditions.
MCF Example 1; the Effect of Media Attrition
In this example we will assume that the standard control vibratory bowl has changed only in that the volume of media is 2” lower due to media attrition. Vibratory bowls having low media volumes are a typical inefficiency found in most vibratory departments. All other variables as determined for the standard control bowl situation will remain the same in this example.
The immediate effect of media attrition is that the column of media above the part’s average surface area is shorter. In this example, the column is 23” tall as compared to 25” tall for the standard control situation.
A secondary effect is that the circumference of the vertical rolling circle is shorter because the diameter of the media in the channel is now only 23”. How does the loss of just two inches of media depth affect the applied media force on the part?
A media mass diameter of 23” means the circumference of the vertical roll has decreased from 78.5” to; (3.14)(23”) = 72.22”. If we assume the same 4 rolls per lap in 60 seconds as noted earlier, the total distance traveled per minute or velocity, has been reduced from 471”/min as follows:
- Radius of media mass = 23” ÷ 2 = 11.5” where; 11.5in ÷ 12in/ft = 0.96ft
- Vertical distance; (4)(72.22”) = 289”
- Horizontal distance traveled = + 157”
- Distance per minute = 446”
- V = 446 in/min ÷ 12 in/ft = 37.17ft/min
- V = 37.17ft/min ÷ 60 sec/min = 0.62ft/sec
The shorter column of media above the average surface area of the part decreases the weight in lbs of media above the part. Calculated as follows:
- (5.33in2)(23”) = 122.59in3
- 59in3 ÷ 1,728in3/ft3 = 0.071ft3media
- = (0.071ft3)(125 lbs/ft3) = 8.88 lbs
2” Media Loss by Attrition: Example No. 1 MCF Calculation
- MCF = (m/g)(v2/r)
- = [8.88 lbs ÷ 32ft/sec2][(0.62 ft2/sec)2÷ 0.96ft]
- = (0.278 lbs/ft/sec2) [(0.38ft2/sec2÷ 0.96ft)]
- = (0.278 lbs/ft/sec2)(0.39ft/sec2)
- MCF = 0.108 lbs of media contact force
2” Media Loss by Attrition: MCF Example 2; the Effect of the Density of the Media Used
In this example we will assume all variables are equivalent to the standard control vibratory bowl situation, except that instead of filling the bowl with HDNA media from Table No. 1 on page 3, the vibratory bowl has been filled with No. 20 media at 85 lbs/ft3. How will this change in media density affect the lbs of media contact force on the average surface area of the part?
The diameter of the media mass remains constant at 25”. Therefore the vertical roll distance remains the same at 78.5”. However the lighter density of the No. 20 media significantly changes the mass weight in pounds, in the media column above the average surface area of the part:
- (5.33in2)(25”) = 133.25in3media above part
- 25in3÷ 1,728in3/ft3 = 0.077ft3media
- (0.077ft3media)(85 lbs/ft3) = 6.55 lbs
Lighter Density Media: Example No. 2 MCF Calculation
- MCF = (m/g)(v2/r)
- = [6.55 lbs ÷ 32ft/sec2][(0.654 ft2/sec)2÷ 1.04ft]
- = (0.205 lbs/ft/sec2) [(0.43ft2/sec2÷ 1.04ft)]
- = (0.205 lbs/ft/sec2)(0.41ft/sec2)
- MCF = 0.084lbs media contact force
MCF Example 3; the Effect of a Change in Mass Velocity
In this example we will return to the Standard Control operating conditions and make one variable change, we will assume the 4 rolls per lap now requires 75 seconds instead of 60 seconds. This is a typical operational change in a vibratory department that can occur when a new processing run of parts is added to the bowl and the new load of parts weighs more than the original load of parts. In such instances the vibratory bowl is now moving more weight and as a result the rolling rate of the mass decreases. How will this change affect the media contact efficiency and therefore the process efficiency of the cycle?
Velocity calculation change as follows:
- Time = 60 sec ÷ 75 sec = 0.80
- Velocity = (471”/min)(0.08) = 377”/min
- 377 in/min ÷ 12 in/ft = 31.42ft/min
- 42ft/min ÷ 60sec/min = 0.524ft/sec
Slower Mass Velocity: Example No. 3 MCF Calculation
- MCF = (m/g)(v2/r)
- = [9.63 lbs ÷ 32ft/sec2][(0.524 ft2/sec)2 ÷ 1.04ft]
- = (0.30 lbs/ft/sec2) [(0.27ft2/sec2÷ 1.04ft)]
- = (0.30 lbs/ft/sec2)(0.26ft/sec2)
- MCF = 0.078lbs of media contact force
MCF Efficiency Comparisons: Standard Control to the Three Examples
For comparison purposes, you will find tabulated in Table No. 2 below the applied media force values for the standard control situation and the three examples just calculated.
Table No. 2; MCF Efficiency Differences
| Example | Variable | lbs Force | Efficiency |
| Standard Control | Optimum Conditions | 0.123 | 100% |
| Example No. 1 | 2” lower media level | 0.108 | 87.8% |
| Example No. 2 | Lighter media density | 0.084 | 68.3% |
| Example No. 3 | Slower mass velocity | 0.078 | 63.4% |
Assuming that the standard control vibratory bowl scenario has been properly vetted and is the optimum operating condition for all similar-sized vibratory bowls in the vibratory room, performing an MCF calculation on each bowl will show how media contact force varies considerably from bowl-to-bowl. What were once often-ignored variables can now be utilized to understand performance inefficiencies and assist in increasing room performance.
Discussion of Results
When setting-up the vibratory department it is critical to establish the most efficient and therefore optimum operational conditions. Obviously this is necessary to ensure that the room is operating at peak efficiency and production throughput can be maximized for consumable cost efficiency.
Within each family of vibratory bowl; as determined by volumetric displacement, will be a set of operational characteristics related to velocity of the mass, depth of media in the channel and density of media used. With the standard control situation established has been established, other bowls having the same volume, can be compared to the standard control bowl using the MCF to determine why processing times vary from bowl-to-bowl.
Previously, vibratory bowl operational conditions were noted by the use of an amplitude gauge. Although useful in capturing the most elemental basics of machine set-up, an amplitude gauge can’t be utilized to determine the performance differences between adjacent machines having varying processing cycle times. This is an aggravatingly-maddening conundrum in situations where the operator is trying to match production cycles and part quality run-after-run in multiple side-by-side machines. By taking just a few measurements to determine mass velocity and the depth of missing media, MCF calculations can now be applied to adjacent machines; of identical volumes, to understand their performance differences.
As mentioned previously, loss of media volume due to attrition, is the single most commonly found performance degradation characteristic in vibratory bowl finishing. Using the MCF in Example 1; showed how significant the loss of just 2” of media depth can make in machine performance. Such a loss is hardly noticed in most vibratory rooms, let alone is it addressed with the timely addition of new media to maintain media level. Yet the degradation of the machine performance would proceed unchecked, run-after-run, until significantly longer times were noticed. The use of the MCF can now be used to calculate answers to previous suppositions reflective of efficiency loss.
Likewise in Example No. 2 the MCF calculation can be used to see how a change in media density will affect processing efficiency. There are situations that require the use of lighter or heavier density media. By using the MCF it is now possible to predict the lengthening or shortening of the vibratory processing cycle as a consequence.
If we consider the bowl’s slower rolling speed; as shown in Example No. 3; the result of a heavier load of parts being placed into the bowl, in the past the only way to measure the bowl’s operation characteristics was by the use of an amplitude gauge. But by using MCF it is now possible to again understand how the processing time will be lengthened, the result of the slower speed.
Table No. 3 below, allows us to view the projected changes in processing time as a result of the three comparison examples.
Table No. 3: Projected Cycle Time Differences Resulting from the Three Previous Examples Correlated to 1 Hour.
| Example Scenario | Efficiency | Time |
| Standard control | 100% | 60 min. |
| Low media level | 87.8% | 68 min. |
| Less dense media | 68.3% | 88 min. |
| Slower rolling rate | 63.4% | 95 min. |
Conclusions
Vibratory finishing efficiency is dependent upon several variables that are most definitely synergistically interrelated. This paper has identified the most critical variables, has described procedures to measure them and has introduced a formula into which the quantitatively measured variables can be substituted to generate qualitatively important performance information.
This paper has additionally shown how the change of one variable such as media depth is synergistically related to distance travelled and then mass velocity. Previously, operators may have supposed an interrelationship between the two but were impotent in capturing and quantifying their suppositions. The introduction of the MCF has given them that tool.
Optimizing performance in the vibratory room is critical for time and consumable efficiency. Attention to operational details are important in maintain this efficiency. It is now possible to not only understand that these variables exist but to also use them to generate a performance advantage or correct inadequacies where and when possible.
William P. Nebiolo is with REM Chemicals, Inc. in Southington, CT. Please visit www.remchem.com.
References
- Lucas, Jim; Live Science Website; “Force, Mass & Acceleration: Newton’s Second Law of Motion;” 2015; www.livescience.com/46560-newton-second-law
- Naval-Technology.com; Image of U.K. Astute Class, SSN Nuclear Submarine; 2002
- Nebiolo, William P.; “An Easily Understood Technique for Measuring Vibratory Bowl Speed and Optimizing Vibratory Bowl Processing Efficiency”; SUR/FIN ‘07; 2007; Cleveland, OH
- Nebiolo, William P.; “A Comparison of the Advantages and Disadvantage of Assorted Mass Finishing Techniques;” SUR/FIN ’09; Louisville, KY
- Nebiolo, William P.; “Estimating the Total Solids Burden During the Waste Water Treatment of Vibratory Finishing Effluents;” SUF/FIN ’11; Rosemont, IL
- Nebiolo, William P.; REM Training Manual Edition No. 9; REM Chemical, Inc.; Southington, CT; Dec. 30, 2014
- subsim.com; website; radio room forum; silent hunter, periscope and antennas fix image
























